JEE Advanced 2019 Paper 2, Question 7
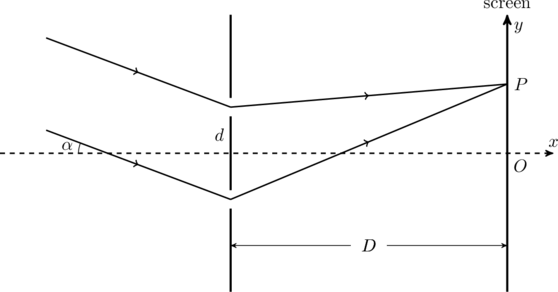
In a Young’s double slit experiment, the slit separation ![]() is 0.3 mm and the screen distance
is 0.3 mm and the screen distance ![]() is 1 m. A parallel beam of light of wavelength 600 nm is incident on the slits at angle
is 1 m. A parallel beam of light of wavelength 600 nm is incident on the slits at angle ![]() as shown in figure. On the screen, the point
as shown in figure. On the screen, the point ![]() is equidistant from the slits and distance
is equidistant from the slits and distance ![]() is 11.0 mm. Which of the following statement(s) is/are correct?
is 11.0 mm. Which of the following statement(s) is/are correct?
- For
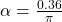 degree, there will be destructive interference at point
degree, there will be destructive interference at point 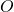 .
. - For
 , there will be constructive interference at point
, there will be constructive interference at point  .
. - For
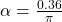 degree, there will be destructive interference at point
degree, there will be destructive interference at point  .
. - Fringe spacing depends on
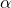 .
.
Solution
This setup is different from the usual double slit experiment in that there is an extra phase shift introduced by the angle of the incident beam, ![]() . This introduces an extra path difference of
. This introduces an extra path difference of ![]() between the two rays. Of course, there is also the path difference
between the two rays. Of course, there is also the path difference ![]() incurred after light passes through the slits. Therefore the two light wavees arrive at the screen with a total path difference of
incurred after light passes through the slits. Therefore the two light wavees arrive at the screen with a total path difference of
(1) ![]()

If we express ![]() in radians, we can approximate
in radians, we can approximate ![]() for small values of
for small values of ![]() . Again, note that it is crucial we represent
. Again, note that it is crucial we represent ![]() in radians when we do this. Furthermore, we have constructive interference when the path difference is an integer multiple of full wavelengths, and it is destructive when it is a half-integer multiple. That is,
in radians when we do this. Furthermore, we have constructive interference when the path difference is an integer multiple of full wavelengths, and it is destructive when it is a half-integer multiple. That is,
(2) 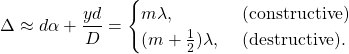
We can now consider each option given in the problem. First we note that ![]() degrees is
degrees is
(3) ![]()
At point ![]() on the screen
on the screen ![]() , and by (2)
, and by (2)
(4) ![]()
which is exactly one wavelength (![]() ), ensuring constructive interference at
), ensuring constructive interference at ![]() . So option (A) is incorrect.
. So option (A) is incorrect.
For ![]() degrees, at point
degrees, at point ![]() (
(![]() ),
),
(5) ![]()
which means we have destructive interference at ![]() . If
. If ![]() were zero, we’d have
were zero, we’d have ![]() , which would also mean destructive interference. So option (B) is incorrect, but option (C) is correct.
, which would also mean destructive interference. So option (B) is incorrect, but option (C) is correct.
Finally, we note that the additional path difference ![]() shifts all the fringes uniformly; the spacing between the fringes is still
shifts all the fringes uniformly; the spacing between the fringes is still
(6) ![]()
which is independent of ![]() . Therefore, option (D) is incorrect.
. Therefore, option (D) is incorrect.